Interpretation of NTO's for SF-TD-DFT

Questions
#1

Hello all,

Im used to NTO’s for TD-DFT where I can interpret the excitation (for example) as a pi to pi* excitation when the homo looks like a pi orbital and the lumo like a pi*, however for NTO’s in SF-TD-DFT i dont get one homo and lumo, but seperate alpha and beta orbital of course. Should I intrepret only the highest occupied alpha to the lowest unoccupied beta?
Thanks in advance and best regards,
Andy

#2

As with spin-conserving TDDFT, you need to look at the amplitudes (NTO singular values), as those are telling you how important is the second (third, etc.) pair of NTOs. Often only a single pair has significant amplitude but that need not always be the case, and more than one significant NTO pair is a signature of unresolvable static correlation in the excited state. See here: [2204.10135] Density Functional Theory for Electronic Excited States

#3

Thank you for your quick response
I use chemcraft for visualizing orbitals:

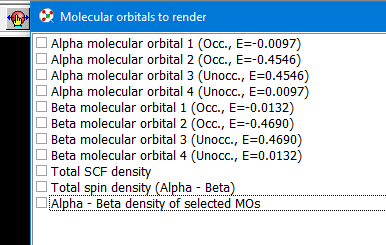
image

and I get 4 (important) NTO’s now, occ a MO 2, unocc a MO 3, occ b MO2, unocc b MO3.
For my molecule I am comparing the TDDFT to the SF-TDDFT orbitals. So my questions remains, how do i interpret the excitation?
Just occ a MO 2 → unocc b MO3. or also occ a MO 2 to unocc a MO 3?
All the best,
Andy

#4

I am not familiar with this program but the interpretation seems clear. The excitation is dominated by (and with approximately equal contributions from) alpha occ 2 → alpha unocc 3 and betat occ 2 → beta unocc 3. (For singlet excitations from a closed-shell ground state, one does expect the amplitudes to be spin-paired in this way.)

If these are indeed the NTO, then I think it must be the case that 0.4546 and 0.4690 are the singular values, not amplitudes, so they should be added directly not squared (i.e., I think they are “lambda**2” in the notation of my review that is cited above). Then 0.4546+0.4690 = 0.9236 or 92% of the norm of the transition density. However, you should check this interpretation against the Q-Chem output file, which spells out more directly what are amplitudes and what are singular values.